Skip to main content

भारतीय रसायन के पिता आचार्य प्रफुल्ल चंद्र रे की जयंती पर व्याख्यान का आयोजन

भारतीय रसायन के पिता आचार्य प्रफुल्ल चंद्र रे की जयंती पर व्याख्यान का आयोजन विज्ञान भारती उदयपुर इकाई एवं बीएन कॉलेज ऑफ फार्मेसी, बीएन विश्वविद्यालय के संयुक्त तत्वावधान में कार्यक्रम सम्पन्न उदयपुर, 2 अगस्त। भारतीय रसायन के पिता आचार्य प्रफुल्ल चंद्र रे की जयंती के अवसर पर विज्ञान भारती उदयपुर इकाई (चित्तौड़ प्रांत) एवं बीएन कॉलेज ऑफ फार्मेसी, बीएन विश्वविद्यालय के संयुक्त तत्वावधान में एक विशेष व्याख्यान का आयोजन किया गया। कार्यक्रम का उद्देश्य आचार्य पी.सी. रे के वैज्ञानिक योगदान एवं उनके देशभक्ति से ओतप्रोत जीवन पर प्रकाश डालना था। ज्ञातव्य है कि भारत की पहली फार्मा कंपनी आचार्य रे ने ही बंगाल केमिकल एंड फार्मास्यूटिकल्स लिमिटेड, कोलकाता में 1901 में प्रारंभ की थी। कार्यक्रम में विज्ञान भारती के उद्देश्य एवं गतिविधियों की जानकारी डॉ. अमित गुप्ता द्वारा दी गई। आचार्य पी.सी. रे के जीवन और कार्यों पर मुख्य व्याख्यान डॉ. लोकेश अग्रवाल द्वारा प्रस्तुत किया गया। उन्होंने बताया कि कैसे आचार्य रे ने विज्ञान को समाज की सेवा का माध्यम बनाया और रसायन विज्ञान में भारत को आत्मनिर्भर बनान...
Post a Comment

Grand canonical ensemble | Statistical mechanics | L-9

Grand canonical ensemble

Grand canonical ensemble

  • Microcanonical ensemble is a collection of independent assemblies in which energy (E), volume (V), and number of particles (N) remain constant.
  • Canonical ensemble is a collection of independent assemblies in which temperature (T), volume (V), and number of particles (N) remain constant.
  • It mean a microcanonical ensemble ⟶ Canonical ensemble, if we ignore the condition E = constant.
  • Therefore the energy exchange takes place in this ensemble.
  • Actually in chemical process, the number of particles N varies and it is very difficult to keep the number of particles constant in various phenomenon like radioactive decay process.
  • Thus grand canonical ensemble is an ensemble in which the exchange of energy as well as the number of particles takes place with the heat reservoir.
  • The grand canonical ensemble is a collection of essentially independent assemblies having the same temperature T, volume V, and a chemical potential µ.

  • Thus grand canonical ensemble is a situation in which we know both the average energy and the average number of particles in assembly, otherwise we don’t know the state of the system.
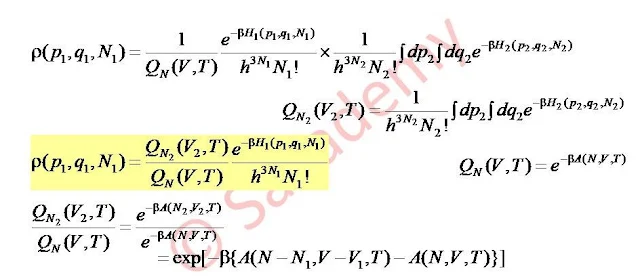
  • The density function in Гspace for grand canonical ensemble = ρ (p, q, N)
  • Here p is momenta, q is coordinate and N is number of particles.
  • To find ρ (p, q, N), we consider the canonical ensemble for a system with particles N, volume V and temperature T.
  • Here we can not take exactly V = constant, so our focus is on a small sub-volume V1 of the system.
  • Let N1 particles be in volume V1, and N2 particles be in volume V2
  • N1 + N2 = N   ⇒   N2 = N - N1    and    V1 + V2 = V   ⇒   V2 = V - V1
  • Also we assume that V2 >> V1 and N2 >> N1
  • If H1 and H2 be the Hamiltonian of the system then
  • The total Hamiltonian of the composite system
  • H (p, q, N) = H1 (p1, q1, N1) + H2 (p2, q2, N2)

Partition function of the system

  • The partition function of the total system
  • Here A (N, V, T) is the Helmholtz free energy.
  • In the last term we consider that the system under consideration to become infinite in size or 0 ≤ N < ∞.
  • For thermodynamic function, we define grand partition function

Average number of particle

  • By ensemble average, the average number of particles

Internal energy

To know more about this topic please click the link https://youtu.be/1dpbmImt8UE
Labels:

Comments

Post a Comment

Popular posts from this blog

Aplanatic points of a spherical refracting surface | Optics | General theory of image formation

Aplanatic points of a spherical refracting surface From Abbe’s sine condition                                  If this ratio is constant for a particular surface, then the surface is known as aplanatic surface . An aplanatic surface is a surface which forms a point image of a point object situated on its axis. The image formed by aplanatic surface is free from optical aberrations. Using sine law in △OPC                                                                            ...(1) Since refraction is taking place from denser to rarer, so from Snell's law              ...
Post a Comment
Read more

Advantage and Disadvantage of Power Electronics

Advantage and Disadvantage of Power Electronics Advantage of Power electronics Power electronics is used in space shuttle power supplies Since there is very low loss in power electronic devices so its efficiency is very high. Power electronic converter systems are highly reliable. Since there is no moving parts in power electronic systems so it has long life and also its maintenance cost is very less. The power electronic systems has fast dynamic response in comparison to electromechanical converter systems. Since the power electronic system has small size and also it has less weight so they occupy less floor space and hence their installation cost is also less. Now these days power equipments are being mostly used, so power semiconductor devices are being produced on a large scale, resulting in lower cost of converter equipment. Power electronics are used in computer and office equipments. It is used in uninterruptible power supplies. Power...
Post a Comment
Read more

गैसों का अणुगति सिद्धान्त तथा आदर्श गैस का दाब | Kinetic theory of gases and Pressure of an ideal gas in Hindi | Thermodynamics

गैसों का अणुगति सिद्धान्त तथा आदर्श गैस का दाब गैसों के गतिज सिद्धान्त की अभिधारणाएं एक गैस अत्यन्त छोटे, अदृश्य एवं पूर्णतः प्रत्यास्थ कणों से मिलकर बनी होती है, जो अणु   कहलाते हैं। एक शुद्ध गैस के सभी अणु समदृश होते हैं तथा ये सभी सम्भव दिशाओं में सभी सम्भव वेग से सतत्‌ रूप से गति करते रहते हैं। गैस जिस पात्र में भरी जाती है, वह उस पात्र की दीवारों पर दाब लगाती है। गैस के अणु किन्हीं दो क्रमागत टक्करों के मध्य सीधी रेखा में गति करते हैं। गैस के अणुओं का आकार किन्हीं दो क्रमागत टक्करों के मध्य तय की गई दूरी की तुलना में अनन्त सूक्ष्म होता है। ये टक्करें तात्क्षणिक होती हैं तथा टक्करों में गतिज ऊर्जा की कोई हानि नहीं होती है। अणु एक दूसरे पर कोई बल नहीं लगाते हैं। वे एक दूसरे पर बल केवल टकराने के दौरान लगाते हैं। इनकी सम्पूर्ण आणविक ऊर्जा, गतिज ऊर्जा होती है। गैस के अणुओं का कुल आयतन, उस पात्र के आयतन, जिसमें यह भरी है कि तुलना में नगण्य होता है। गैस में अन्तर-आणविक दूरी बहुत अधिक होती है, जिससे कि गैस के अणु उसके लिए उपलब्ध सम्पूर्ण स्थान में मुक...
Post a Comment
Read more